
Backtesting in Excel
The article is about how to do Backtesting in Microsoft Excel, it’s modeling refers to a predictive model’s testing using historical data.
Home » Using NumXL » Page 2
On this page, find free resources on using NumXL functions and wizards in Microsoft Excel for data analysis and forecasting.

The article is about how to do Backtesting in Microsoft Excel, it’s modeling refers to a predictive model’s testing using historical data.
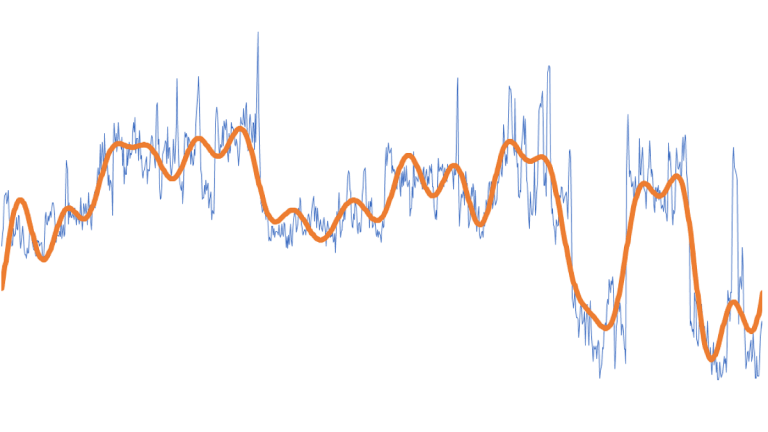
This issue addresses how to use Fourier Transform to filter a data signal using only K frequency components with the highest amplitudes.

Over the past few weeks, several users reported various problems with NumXL toolbar in Office 365 on Windows 10. After a thorough investigation and long troubleshooting sessions, we have realized that a recent Microsoft Windows Cumulative update (KB4511553) released back in August 2019, has broken parts of our VBA code in the NumXL toolbar.
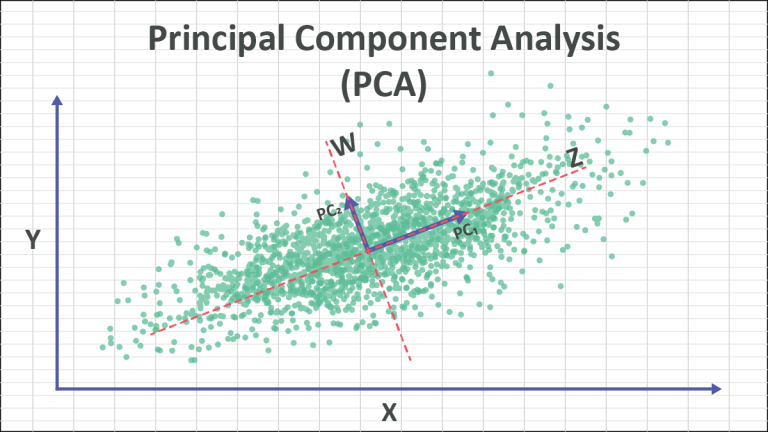
Principal Component Analysis (PCA) simplifies modeling by transforming correlated variables into uncorrelated factors using NumXL in Excel.
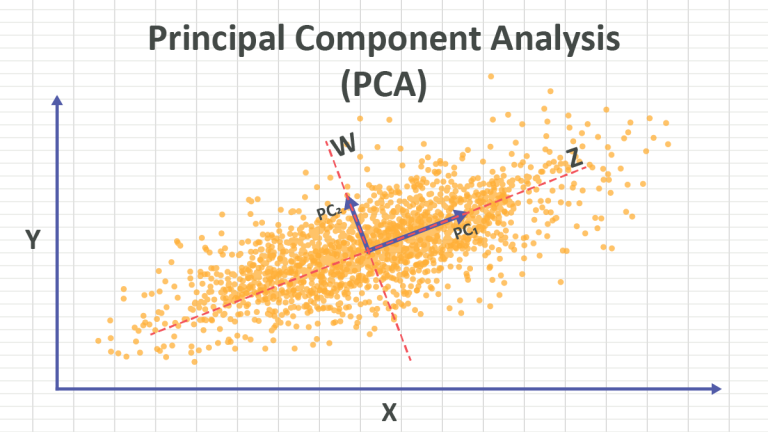
This article continues the PCA in Excel series, examining dimension reduction from 5 PCs to 3 PCs without significant information loss.
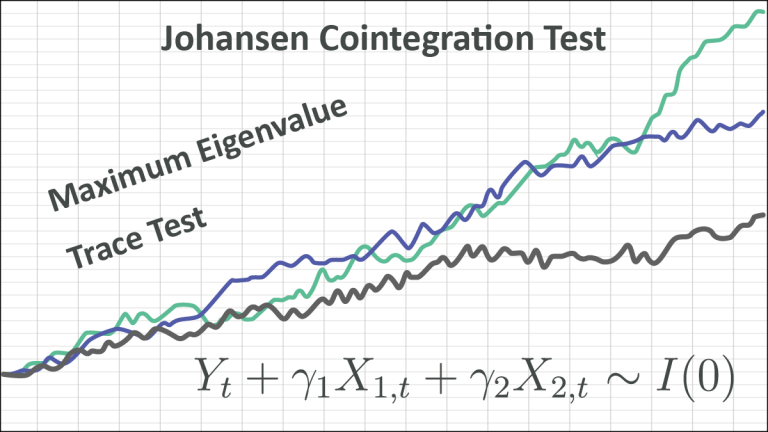
Step-by-step guide to perform Johansen Cointegration Test and determine cointegration of non-stationary time series variables.
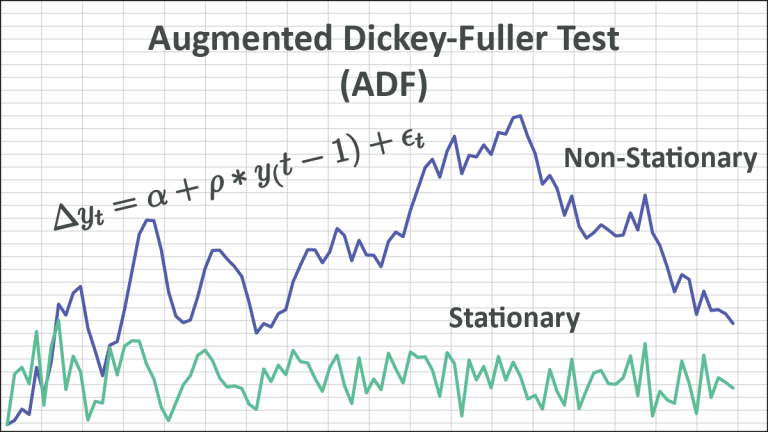
Discover how the ADF Stationary Test checks for stationarity in time series data. NumXL’s blog provides a detailed explanation and examples.

There are several block sites that are using our name NumXL and offering a free cracked version of our software. Those sites are not affiliated with NumXL and often times their downloads contain malware and viruses that can harm your machine.

We are excited to announce a couple of new developments on the NumXL support front: (1) We revamped the ‘Getting Started’ user’s guide, and (2) We are pooling all the NumXL tutorial videos and making them available on a single Youtube channel.

This week, we’ll take the prior discussion further and develop an understanding of autoregressive conditional heteroskedasticity (ARCH) volatility modeling. Why do we care? Volatility cannot be directly
© 2025 Spider Financial Corp | Terms & Conditions | Disclaimer | Privacy Policy | Trademarks & Copyrights